CHAPTER LV.
 

73
 


 

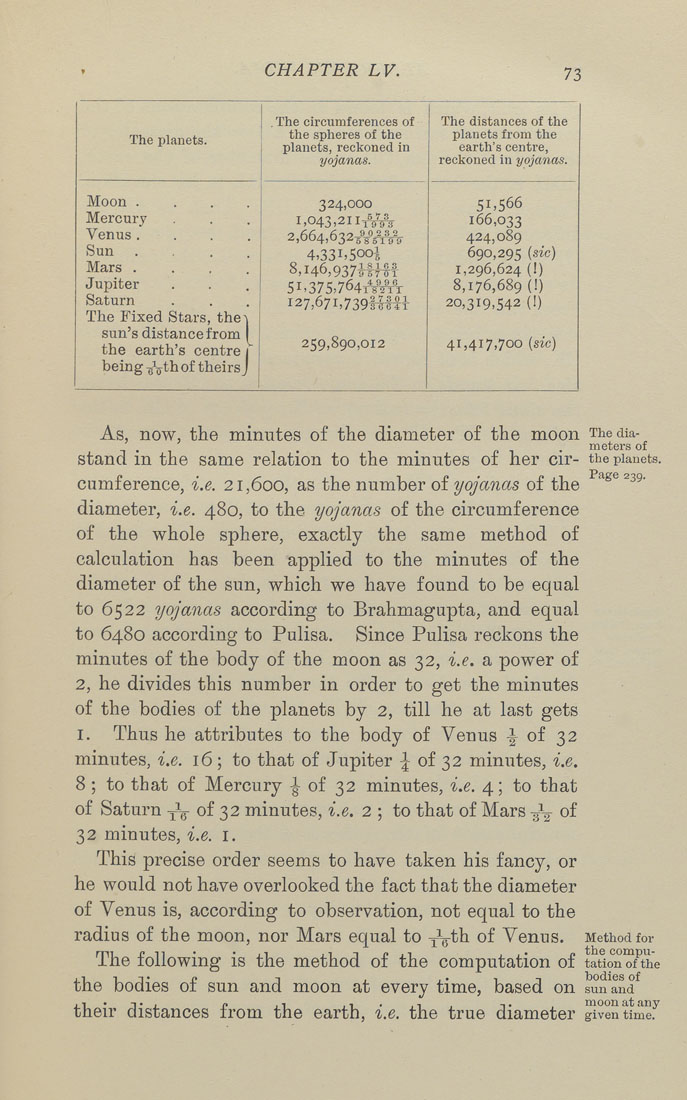
. The circumferences of
 

The distances of the
 

The planets.
 

the spheres of the
 

planets from the
 

planets, reckoned in
 

earth's centre.
 


 

yojanas.
 

reckoned in yojanas.
 

Moon ....
 

324,000
 

51,566
 

Mercury
 

1,043,21 ItVVV
 

166,033
 

Venus ....
 

2,664,6323VAy9
 

424,089
 

Sun     ....
 

4,331,500-1
 

690,295 (sic)
 

Mars  ....
 

8,i46,937^ffM-
 

1,296,624 (!)
 

Jupiter
 

5i,375,764i¥A-''t
 

8,176,689 (!)
 

Saturn
 

i27,67i,739Htlf
 

20,319,542 (!)
 

The Fixed Stars, the^
sun's distance from 1
the earth's centre i
being -g^^th of theirs j
 


 


 


 


 

259,890,012
 

41,417,700 (sic)
 


 


 

As, now, the minutes of the diameter of the moon
stand in the same relation to the minutes of her cir¬
cumference, i.e. 21,600, as the number oi yojanas of the
diameter, i.e. 480, to the yojanas of the circumference
of the whole sphere, exactly the same method of
calculation has been applied to the minutes of the
diameter of the sun, which we have found to be equal
to 6522 yojancts according to Brahmagupta, and equal
to 6480 according to Pulisa. Since Pulisa reckons the
minutes of the body of the moon as 32, i.e. a power of
2, he divides this number in order to get the minutes
of the bodies of the planets by 2, till he at last gets
I. Thus he attributes to the body of Venus -| of 32
minutes, i.e. 16 ; to that of Jupiter ^ of 32 minutes, i.e.
8 ; to that of Mercury ^ of 32 minutes, i.e. 4; to that
of Saturn yL of 32 minutes, i.e. 2 ; to that of Mars -^^ of
32 minutes, i.e. i.

This precise order seems to have taken his fancy, or
he would not have overlooked the fact that the diameter
of Venus is, according to observation, not equal to the
radius of the moon, nor Mars equal to yV^^ of Venus.

The following is the method of the computation of
the bodies of sun and moon at every time, based on
their distances from the earth, i.e. the true diameter
 

The dia¬
meters of
the planets.
Page 239.
 

Method for
the compu¬
tation of the
bodies of
sun and
moon at any
given time.